On the incapability of inertial forces as a means of repeated self-propulsion of an object in a vacuum

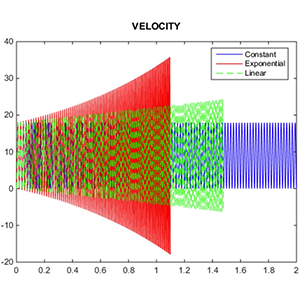
This paper deals with the controversial topic of "inertial propulsion". It discusses in depth the limits of possible upward vertical motion of a vehicle equipped with a couple of contra-rotating masses. Although inertial forces are internal forces in the system, they can cause the center of mass to move upwards up to a certain distance (first cycle of motion), provided the vehicle is initially supported, for example on the ground surface. To make the matter easier to understand, we also contrast it with the operation of the mass-spring system. In contrast to many patents which claim that the key ingredient to achieve a supposed “net thrust” is to invent a way to achieve nonzero impulse of the inertial forces in each rotation of the masses, this paper shows that the main reason of the motion is the initial velocity of the center of mass which is associated with the initial orientation of the rotating arms carrying the masses. Moreover, it is shown that the pattern of the angular velocity only affects the vehicle’s velocity while the position of the vehicle slightly oscillates around the standard position of the center of mass which always performs the motion of a mass particle in vertical shot. In the end, it was shown that non-zero thrust of the inertial forces per revolution is possible in many ways, but in no case ensures repeated propulsion of the vehicle in a vacuum, the realization of which requires either intermediate support conditions or reaction forces at the time when the maximum travel in each movement cycle is completed.